An international team of scientists including researchers from the UW’s Faculty of Physics, the Laboratoire Matière et Systèmes Complexes, and the Institut des Sciences de la Terre d’Orléans has described how loops, important for the stability of transport networks, occur in those found in nature. The researchers observed that when one branch of the network reaches the boundaries of the system, the interactions between the branches change dramatically.
Previously repulsive branches, begin to attract each other, leading to the sudden formation of loops. The findings were published in the “Proceedings of the National Academy of Sciences” journal.
Many examples of transport networks can be found in nature, from the network of blood vessels in our bodies to electrical discharges in a thunderstorm.
“Such networks take on a variety of shapes,” Stanislaw Żukowski, a doctoral candidate from the University of Warsaw and Université Paris Cité and lead author of the paper, explained, adding: “They can have a tree-like geometry, in which parts of the network merely branch and repel each other during growth. In other cases, where branches attract each other during growth and reconnect, we are dealing with looped structures.”
Networks with multiple loops are widespread in living organisms, where they serve to actively transport oxygen or nutrients and carry away metabolic products. An important advantage of looped networks is that they are less susceptible to damage – in networks without loops, the destruction of one branch can cut off all branches connected to it, whereas in networks with loops there are always other connections to the rest of the system. Recently, researchers from the UW’s Faculty of Physics described the mechanism responsible for the stability of already existing loops. However, the dynamic process leading to their formation remained incompletely explained.
How are loops formed?
Many transport networks grow in response to a diffusion field, such as the concentration of a substance, the pressure in the system or the electric potential. The fluxes of such a field are much more easily transported through the branches of the network than through the surrounding medium. This affects the distribution of the field in space – lightning conductors attract electrical discharges precisely because they have less resistance than the surrounding air. The large difference in resistance between the grid and the medium leads to competition and repulsion between the branches.
However, the attraction of branches in growing networks, leading to the formation of loops, remained undescribed for a long time. The first attempt to understand the formation of loops in such systems was made a few years ago by Prof. Piotr Szymczak’s group at the UW’s Faculty of Physics.
“We showed that a small difference in resistance between the lattice and the medium can lead to attraction between growing branches and the formation of loops,” Piotr Szymczak said.
The work led to a joint project, in the form of a combined doctoral thesis by Stanisław Żukowski, carried out in Prof Piotr Szymczak’s group and the group of Annemiek Cornelissen, a researcher at the Laboratoire Matière et Systèmes Complexes.
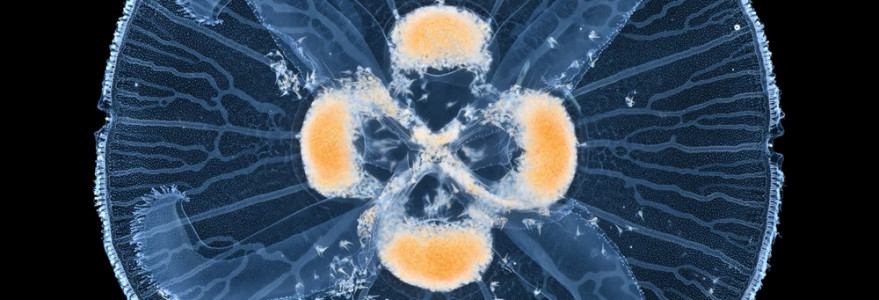
“In our laboratory, we study the morphogenesis of the jellyfish digestive system network. It’s a beautiful example of a transport network with many loops,” Annemiek Cornelissen said.
“When I saw Annemiek’s presentation at a conference in Cambridge a few years ago, I immediately thought that our models could be applied to describe the growth of channels in jellyfish,” Prof. Szymczak added.
Through the stomach to the loop
“The formation of loops when one of the branches reaches the boundaries of the system – a phenomenon we describe in our latest publication – was indeed first noticed in the channel network of the jellyfish’s digestive system” Stanislaw Żukowski said, adding: “By analysing the development of these channels over time, I noticed that when one of them connects to the jellyfish’s stomach – the boundary of the system – the shorter channels are immediately attracted to their longer neighbour and form loops.”
The scientists observed the same phenomenon in gypsum fracture dissolution experiments conducted at the University of Warsaw by Florian Osselin, in the so-called Saffman-Taylor experiment, in which the boundary between two fluids is unstable and transforms into finger-like patterns. They also encountered it in the literature on electrical discharges.
“The wealth of systems in which we discovered very similar dynamics reinforced our belief that there must be a simple, physical explanation for this phenomenon,” Annemiek Cornelissen said.
In their publication, the researchers presented a model that describes the interactions between branches. They focused on how these interactions change when one of the branches approaches the boundary of the system and a breakthrough occurs.
“Then, the competition and repulsion between branches disappears and attraction appears,” Stéphane Douady explained, adding: “This inevitably leads to the formation of loops.”
Loops near the puncture
“Our model predicts that attraction between adjacent branches after a puncture occurs regardless of the geometry of the network or the difference in resistance between the network and the surrounding medium,” Prof. Piotr Szymczak said, adding: “In particular, we have shown that loops in the vicinity of a puncture can form in systems with a very large difference in resistance, which was previously thought to be impossible, but explains why this phenomenon is so prevalent in physical and biological systems.”
“In cases where the growth mechanisms have not yet been elucidated, observing the phenomenon we have described will be a strong indication that the dynamics of the system is controlled by diffusion fluxes. We are extremely curious to see in which other systems we will observe loop formation after breakthrough.” Stanislaw Żukowski added.